This is my favorite tweet of the moment, courtesy of Dan Meyer:
So glad the @nytimes found an answer to their question from 2012, “Is Algebra Necessary?” [h/t a commenter] pic.twitter.com/QQoC3w1BWE
— Dan Meyer (@ddmeyer) December 30, 2014
Back in July 2012, the New York Times published a terrible, ignorant, anti-intellectualism-spouted-by-a-so-called-intellectual opinion piece titled Is Algebra Necessary? It was penned by Andrew Hacker, an emeritus professor of political science (that should be your first warning) at Queens College, City University of New York. His thesis was that making math mandatory reduces our talent pool by discouraging “otherwise talented students who are impeded by algebra, to say nothing of calculus and trigonometry.” The horror!
Click the image to read the original article.
To their credit, the Times published a couple of follow-up pieces soon afterward: In Defense of Algebra and N Ways to Apply Algebra to the New York Times. However, they only truly atoned for their math sins a couple of days ago in a piece about how much money the movie The Interview made:
While Sony didn’t say how much of the $15 million made came from rentals and sales, anyone with eighth-grade algebra would realize that there’s enough information to figure it out. In these two paragraphs, you have two variables and two linear equations, which means you don’t need Sony to tell you how much of that $15 million came from sales and how much came from rentals.
To make your life easier, I’ve done the math for you:

So to answer the New York Times’ question, “Is algebra necessary?”, the answer is “Yes, and more often than you might think.”

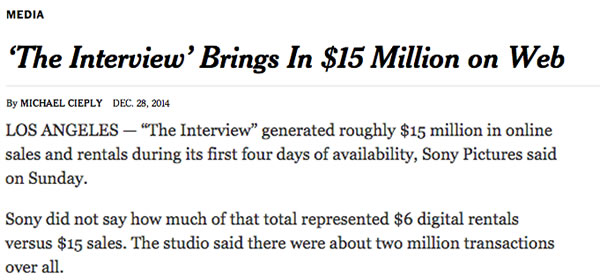

31 replies on “The New York Times unintentionally answered their question, “Is Algebra Necessary?””
[…] This awesome post that a friend of mine linked to on Facebook this morning has a great real world application of simple algebra. […]
Please explain equation 3. I really don’t gfet it and its throwing me off.
Good point, but please remember the condition number. Given round off on significant figures (assuming 2 not 1), the answers get a little more complicated, ranging from .96 million rentals to 2.4 million rentals and 22,000 to 640,000 sales.
Curt
In no way is that the only solution. The New York Times is right, there isn’t enough information to figure out how many rentals vs. sales there were. For example, there could have been 100 sales, and 2,499,750 rentals.
And by ‘right’, I mean ‘wrong’!
[…] not to explore the virtues or flaws with Professor Hacker’s arguments, but to point out what many bloggers have recently observed: that the New York Times answered their own question last week in […]
[…] Eugene Stern, who blogs at Sense Made Here, describes himself as “interested in math and modeling and how they are used and taught in real life”. A friend of his pointed on Facebook to my recent post in which I did the math that the New York Times didn’t do when reporting on sa… […]
Curtis: You’re right; I just wanted to avoid the confusion that might arise if I wrote “6” and “15” on one side, and “2 million” instead of “2 000 000” on the other. I’ve seen less math-y people get tripped up by that.
tk: Equation 3 is equation 1, multiplied by 6.
Alan and Curt,
Keep in mind that there were only 2M
total transactions e.g. total number of rentals plus total number of sales = 2M.
Both of your counterpoints seem to overlook this known variable. Anyone who actually knows alegebra, knows that to solve mult-variable problems then you’ve got to eork solve multiple equations concurrently. In this case, there are actually 2 equations at play which need to be solved.
1st being: ( r+s=2,000,000) also stated as (r= 2,000,000-s)
2nd being: 6r + 15s = 15,000,000
That said, we can now combine these 2 equations as one to get the solution. Since we know what r is in terms of s the final equation should now be:
6(2,000,000-s) +15s = 15,000,000 or simply s=333,333.33333…
once we know s we just plug back in to 1st equation.
r=2,000,000-333,333.3333…
r=1,666,666.6666…
In short, Andrew is correct. The world clearly needs algebra and math in general…
Alan: You’re missing that there were 2 million transactions overall. Your answer has 2.5 million.
Sorry, I meant to say that Joey is right.
There is enough info to solve this problem.
It seems the real problem is that our society lacks the critical thinking skills to contextualize information and make educated inferences.
-Adam
Let the algebra lessons begin!
No Alan, there could not have been ” 100 sales, and 2,499,750 rentals” because that doesn’t add up to two million transactions. That’s what equation 1 states.
Brad — you’re wrong. There may have been 2.49999 million transactions, as that would round down to 2 million. I disagree with this post entirely. I don’t think the Times is at fault at all. Sony did not provide the breakdown, and the writer knew that “about” 2 million could mean anything from 1.5 million to 2.499 million, and therefore correctly concluded that the math could not be done with any level of accuracy.
I’m sure plenty of people at the times could figure out the math. What we have here is journalists doing good journalism. As a journalist you never want to make an assertion unless it’s documented or backed up by quotation. I would never run a number in a newspaper that was gathered from variables that included adjectives like “roughly” before them. They were just covering their asses.
The numbers were given approximately. Considering that the value of “about 2 million” can be anything from 1,500,000 up to 2,499,999, and “roughly 15 million” should be between 14,500,000 and 15,499,999, we can find the minimum and maximum values of variables r and s from the following two sets of equations:
1st set: r+s=1,500,000 and 6r+15s=15,499,999 => gives r=777,778 and s=722,222.
2nd set: r+s=2,499,999 and 6r+15s=14,500,000 => gives r=2,555,554 and s=-55,555. Obviously s cannot be less than zero, so after that correction, the conclusion should be that the number of rentals was between .77 million and 2.5 million, and the number of sales was between 0 and .78 million.
This completely proves and dis-proves why we need algebra.
By the numbers, yes, we can calculate almost exactly how many rentals and how many purchases were made. Congratulations – mathematically, you’ve solved the problem! But to the rest of the world, you’ve only generated another statistic.
So what can we do with this statistic? Not much. How many people watched each one of those 1.6 million rentals? What’s the average viewing data? How many views have those sales garnered? At $15 million, how much of a bath is Sony still taking on this film?
More households watched the 2014 US Open (GOLF) then saw The Interview.
A quarter of the New York City population could have seen The Interview.
The 2014 INDY 500 was THREE TIMES more popular then The Interview.
Those statistics, and bits of information, the context, cannot be pulled purely from the numbers, no matter how hard you try.
And this is the issue with knowing Algebra. Yes, it’s great to solve problem where you know all the numbers. But you often don’t have hard numbers. And even when you do, you still need to work with the context to understand what the numbers really say.
Guys you maybe all have a point. Now we could involve probabilities and statistics based on other over hyped shit movies OR the more prudent option might be to just wait a few weeks for the next sony hack and we can get the exact numbers.
“The numbers were given approximately. Considering that the value of “about 2 million” can be anything from 1,500,000 up to 2,499,999, and “roughly 15 million” should be between 14,500,000 and 15,499,999”
Except that you always round up for sales figures, never down.
if they hit 2.5 they would say 2.5
its more like 1.9-2.0 or they would have said a little over 2 million
as far as transactions you want to maximize that number again with 15 at the cap
but unless you want to go into statistics, id say that the numbers fall within 1 sd on the normal curve for accuracy.
Mark s, I think the ratio of rentals to purchases is valuable info, especially since this movie was pulled from mainstream theaters. People might have been more likely to buy, to ensure that they could re watch the movie if it isn’t available in the future. I read that article on the interview and wondered what the ratio was and how it compared with the average for other releases. Sadly, I was too lazy and unpracticed to realize I could do it. But I did stop mid article to solve it (using substitution), continued on to read the more eloquent solution, and also read in the comments about the ranges of values depending on how the stats were rounded. I write code and queries all day and rarely do math anymore, so this is a sad realization, that it’s so rusty for me. We need more algebra and stats in school, and as adults have to force ourselves to keep up with it and stay fresh. Even if the nyt was just being responsible by not figuring out the breakdown, thanks for writing this, it was a great reminder of how knowledge helps us to succeed.
It doesn’t really matter whether the math here is correct or not.
If it is, some of the people claiming that it isn’t could do with some algebra lessons to stop themselves making an ass of themselves.
If it isn’t, Joey Devilla similarly could do with some algebra lessons.
In any case, I could use some algebra lessons myself because I can’t tell if the math’s correct. So either way, arguments in favour of algebra lessons win.
It’s interesting to me that there are people vehemently arguing against the blind application of mathematical rules without thinking things through first, who nevertheless think that 1.5 million sales could be honestly reported as “about 2 million” because of rounding rules.
I think this was a very clever way for a math teacher (and Dan Meyer is a brilliant teacher!) to show his students how something that was claiming to have no answer could actually be solved with Algebra! (Whether it is exactly precise or not!) I would rather have solved this problem in class, taken straight from the headlines, than been given a system of equations with two variables and been told to “solve”. The NY Times problem is much more interesting! 🙂 Good job, Dan!
Use a combination of algebra and common sense.
Some people are claiming that “about 2 million” can technically mean anywhere from 1.5m to 2.4999m sales.
Mathematically, you may be correct based on rounding rules, but if you just think about it, clearly Sony wouldn’t say “about 2 million” is they were off by 25% either way. It’s fairly safe to assume that we’re probably within a much smaller window on either side of 2M.
Is there enough information here to give an exact number of sales and rentals? Of course not, and if you truly think that’s what DeVilla is suggesting, you’re missing the point. The point is: The numbers were there to make a general statement about approximate sales and rental numbers, and the same newspaper that told us algebra wasn’t necessary just gave a perfect example where knowing algebra answered a question (albeit with an approximation) that was otherwise left unanswered.
Don’t know if anyone will see this since it’s been so long — HOWEVER, algebra is so totally not necessary to solve this problem I cannot imagine doing it this way. I used to give my incoming high school algebra students an equivalent problem on the first day of school and command them to do it without algebra. Most could. Only a math teacher would do this problem with algebra — and this particular math teacher would not use algebra. Your argument against Hacker’s original argument fails. In fact, algebra is not necessary at all.
Matt and Curt touched on assumptions. It seems to me that, somewhere way back, we used to state assumptions first and then follow through on logic afterwards. State the assumption that there were exactly 2,000,000 sales and the initial algebra works. State the assumption that 2 million has only one significant figure and other logic prevails. Stating assumptions allows us to see that the math and interpretation of the problem jive. In the absence of stated assumptions, there are probably generally accepted assumptions.
http://wolframalpha.com/input/?i=6a%2B15b%3D15*10%5E6+AND+a%2Bb%3D2*10%5E6&x=9&y=5
David Newell: “In the absence of stated assumptions, there are probably generally accepted assumptions.”
This should be a bumper sticker. We are all so often tripped up by our unconscious “generally accepted” assumptions.
Alan,
There is definitely enough information to solve this. When you solve a system of linear equations, you are solving for (if there is one) the point at which the 2 lines intersect: the point at which BOTH equations are satisfied. Therefore, there IS only ONE solution here (in this case at least, sometimes they dont intersect). You have 2 variables, so there are 2 equations, which is all you need to find the values of each variable that satisfy both equations simultaneously.
I checked the math a different way, and it is correct.
Instead of doing the system of equations this way as OP did, or as a matrix, you instead can just solve each starting equation for one of the variables, and get the value for that variable. That allows you to then find the other. Here is my math:
15s + 6r = $15,000,000 and s + r = 2,000,000
If s + r = 2,000,000 ….. then r = 2,000,000 – s .
If 15s + 6r = $15,000,000 …. then r also = 15,000,000 – 15s / 6
Therefore, 2,000,000 – s = 15,000,000 – 15s / 6
* 6 *6
12,000,000 – 6s = 15,000,000 – 15s
+ 6s +6s
12,000,000 = 15,000,000 – 9s
-15,000,000 -15,000,000
so, -3,000,000 = -9s
/-9 /-9
333,333 = s
Once you have that, you can solve for r. The OPs math is correct.
Julie, who likely will never see this comment…
How is this solved without any algebra? Im interested to see it.
Substitution is an algebra concept.
Linear combination is also an algebra concept.
Graphing both equations requires algebra knowledge…
What method would your students use to solve this without algebra? Or what method would you yourself use, apparently?